Maths week 8 graded assignment Complete Solutions Are Discussed In This Blog We Hope This Might Help You All In Matching Answers . Or For Some Others Reasons Not Able To Complete Graded Assignments
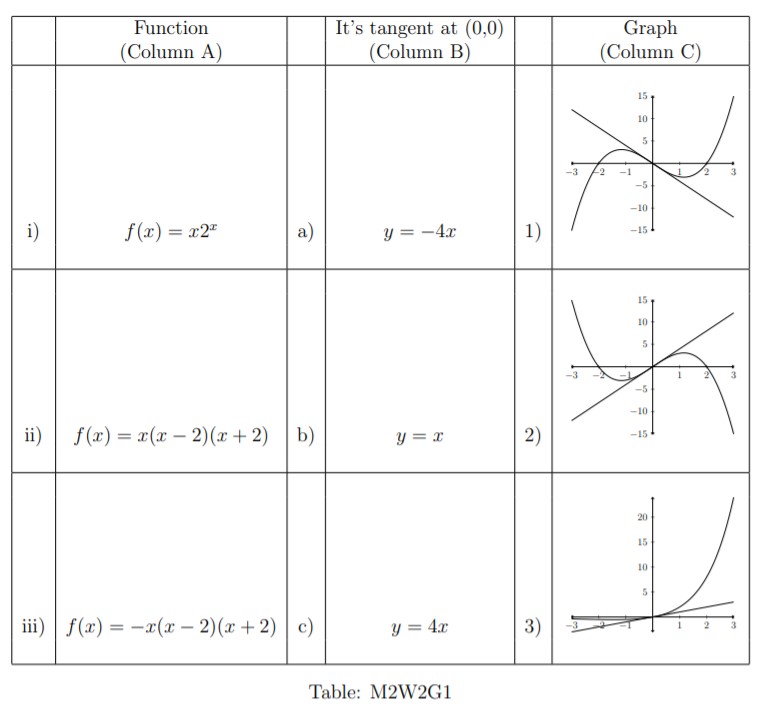
1.Match the given functions in Column A with the equations of their tangents at the origin (0,0)(0,0) in column B and the plotted graphs and the tangents in Column C, given in Table M2W2G1.
No, the answer is incorrect.
Score: 0
Accepted Answers:
2.Consider the following two functions �(�)f(x) and �(�)g(x).
�(�)={�3−9��(�−3)if �≠0,33if �=00if �=3f(x)=⎩⎨⎧x(x−3)x3−9xif x=0,33if x=00if x=3
�(�)={∣�∣if �≤2⌊�⌋if �>2g(x)={∣x∣⌊x⌋if x≤2if x>2
Choose the set of correct options.
No, the answer is incorrect.
Score: 0
Accepted Answers:
3.
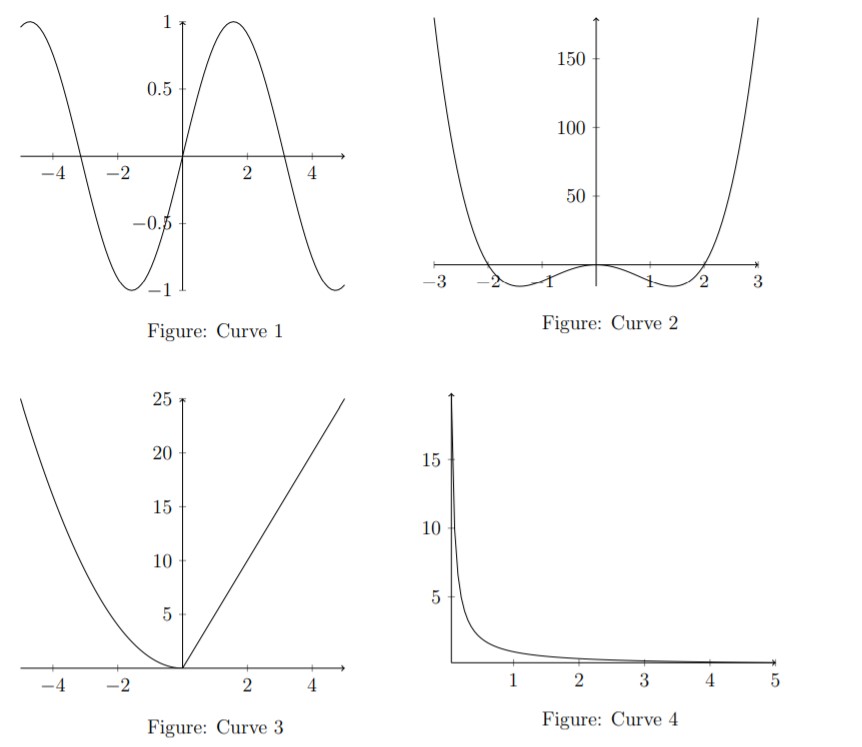
Choose the set of correct options from the below.
No, the answer is incorrect.
Score: 0
Accepted Answers:
4.Choose the set of correct options considering the function given below:
�(�)={��� ��if �≠0,1if �=0f(x)={xsin x1if x=0,if x=0
No, the answer is incorrect.
Score: 0
Accepted Answers:
5. Let �f be a polynomial of degree 5, which is given by
�(�)=�5�5+�4�4+�3�3+�2�2+�1�+�0.f(x)=a5x5+a4x4+a3x3+a2x2+a1x+a0.
Let �′(�)f′(b) denote the derivative of �f at �=�x=b. Choose the set of correct options.
No, the answer is incorrect.
Score: 0
Accepted Answers:
6. Let �f be a differentiable function at �=3x=3. The tangent line to the graph of the function �f at the point (3,0)(3,0), passes through the point (5,4)(5,4). What will be the value of �′(3)f′(3)?
No, the answer is incorrect.
Score: 0
Accepted Answers:
7. Let �f and �g be two functions which are differentiable at each �∈�x∈R. Suppose that, �(�)=�(�2+5�)f(x)=g(x2+5x), and �′(0)=10f′(0)=10. Find the value of �′(0)g′(0).
No, the answer is incorrect.
Score: 0
Accepted Answers:
8. Consider a function �:�→�f:R→R defined as �(�)={sin12�+�sin�15�3if �≠0,�if �=0.f(x)={15x3sin12x+AsinxBif x=0,if x=0. If �(�)f(x) is continuous at �=0x=0 , then find the value of 90�−�90B−A
No, the answer is incorrect.
Score: 0
Accepted Answers:
9. The distance (in meters) traveled by a car after �t minutes is given by the function �(�)=�(6�3+5�2+8�+5)d(t)=g(6t3+5t2+8t+5) , where �g is a differentiable function with domain �R . Find the instantaneous speed of the car after 5 min, where �′(920)=2g′(920)=2
No, the answer is incorrect.
Score: 0
Accepted Answers:
10. Consider the following two functions
�:�→�p:R→R
�(�)={6�(�−5)−6�−5if 0≤�<5,6�=56(�2−25)1ln(�−5)if �>5p(t)=⎩⎨⎧t−56e(t−5)−666(t2−25)ln(t−5)1if 0≤t<5,t=5if t>5
and
�:�→�q:R→R
�(�)=∣�(�−5)(�−8)∣q(t)=∣t(t−5)(t−8)∣ and the following statements (a function is said to be continuous (respectively differentiable) if it is continuous (respectively differentiable) at all the points in the domain of the function).
Statement P:Statement P: Both the functions �(�)p(t) and �(�)q(t) are continuous.
Statement Q:Statement Q: Both the functions �(�)p(t) and �(�)q(t) are not differentiable.
Statement R:Statement R: �(�)p(t) is continuous, �(�)q(t) is differentiable.
Statement S:Statement S: �(�)q(t) is continuous, �(�)p(t) is not differentiable.
Statement T:Statement T: Neither �(�)p(t) nor �(�)q(t) is continuous.
Find the number of correct statements.
No, the answer is incorrect.
Score: 0
Accepted Answers:
�:�→�p:R→R
�(�)={8�(�−5)−8�−5if 0≤�<5,8�=58(�2−25)1ln(�−5)if �>5p(t)=⎩⎨⎧t−58e(t−5)−888(t2−25)ln(t−5)1if 0≤t<5,t=5if t>5
If linear function ��(�)=��+�Lp(t)=At+B denotes the best linear approximation of the function �(�)p(t) at the point �=1t=1, find the value of−8�−4−1(�+�)e−4−1−8(A+B)
No, the answer is incorrect.
Score: 0
Accepted Answers:
12. Consider the following function
�:�→��(�)=∣�(�−2)(�−8)∣.q:R→Rq(t)=∣t(t−2)(t−8)∣.
If �m is slope of the tangent of the function �(�)q(t) at point �=32t=23, find the value �−274m−427
No, the answer is incorrect.
Score: 0
Accepted Answers:
The population of a bacteria culture of type A in laboratory conditions is known to be a function of time of the form
�:�→�p:R→R
�(�)={�3−27�−3if 0≤�<3,27�=31�81(�−3)(�27�−�81)if �>3p(t)=⎩⎨⎧t−3t3−2727e81(t−3)1(e27t−e81)if 0≤t<3,t=3if t>3
where �(�)p(t) represents the population (in lakhs) and �t represents the time (in minutes). The population of a bacteria culture of type B in laboratory conditions is known to be a function of time of the forM
�:�→�q:R→R
�(�)={(5�−9)1�−2if 0≤�<2,�4�=2��+2−�4�−2if �>2q(t)=⎩⎨⎧(5t−9)t−21e4t−2et+2−e4if 0≤t<2,t=2if t>2
where �(�)q(t) represents the population (in lakhs) and �t represents the time (in minutes).
Using the information above, answer questions 13,14 and 15.
Statement P:Statement P: Both the functions �(�)p(t) and �(�)q(t) are continuous.
Statement Q:Statement Q: �(�)p(t) is continuous, but �(�)q(t) is not.
Statement R:Statement R: �(�)q(t) is continuous, but �(�)p(t) is not.
Statement S:Statement S: Neither �(�)p(t) nor �(�)q(t) is continuous.
Find the number of the correct statements.